Dissipation of energy in closed systems

Learn in this article why, in thermodynamic processes with dissipation of energy, the pressure-volume work of the gas does not correspond to the work done by the system.

Pressure-volume work (displacement work)

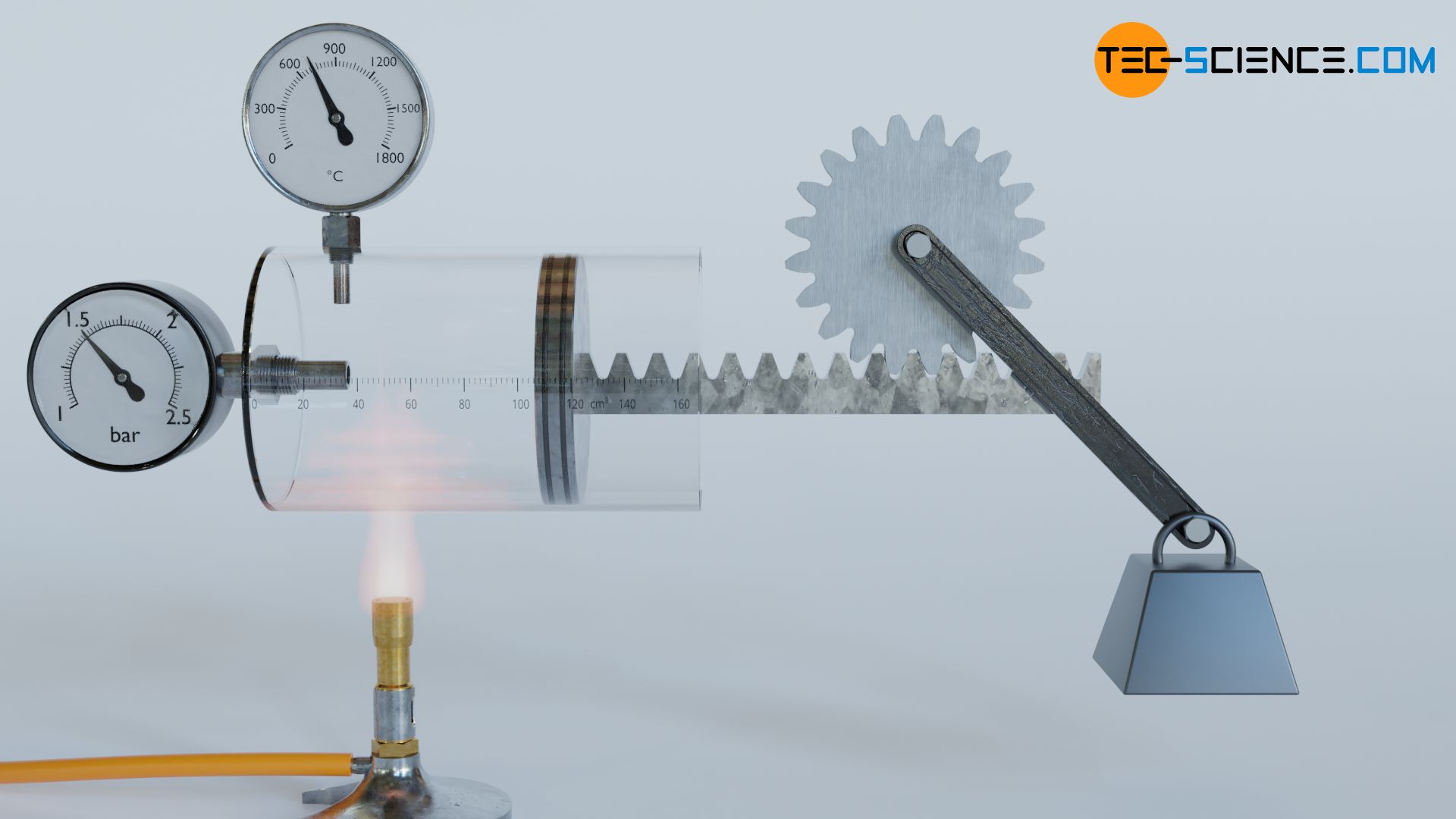
In the article Concept of pressure-volume work it was explained in detail that the work done by the gas or on the gas in a thermodynamic process corresponds to the area under the curve in a volume-pressure diagram. For this purpose, we imagine a gas in a cylinder, which is closed by a piston. By heating the gas, a weight is lifted via a rack and pinion.

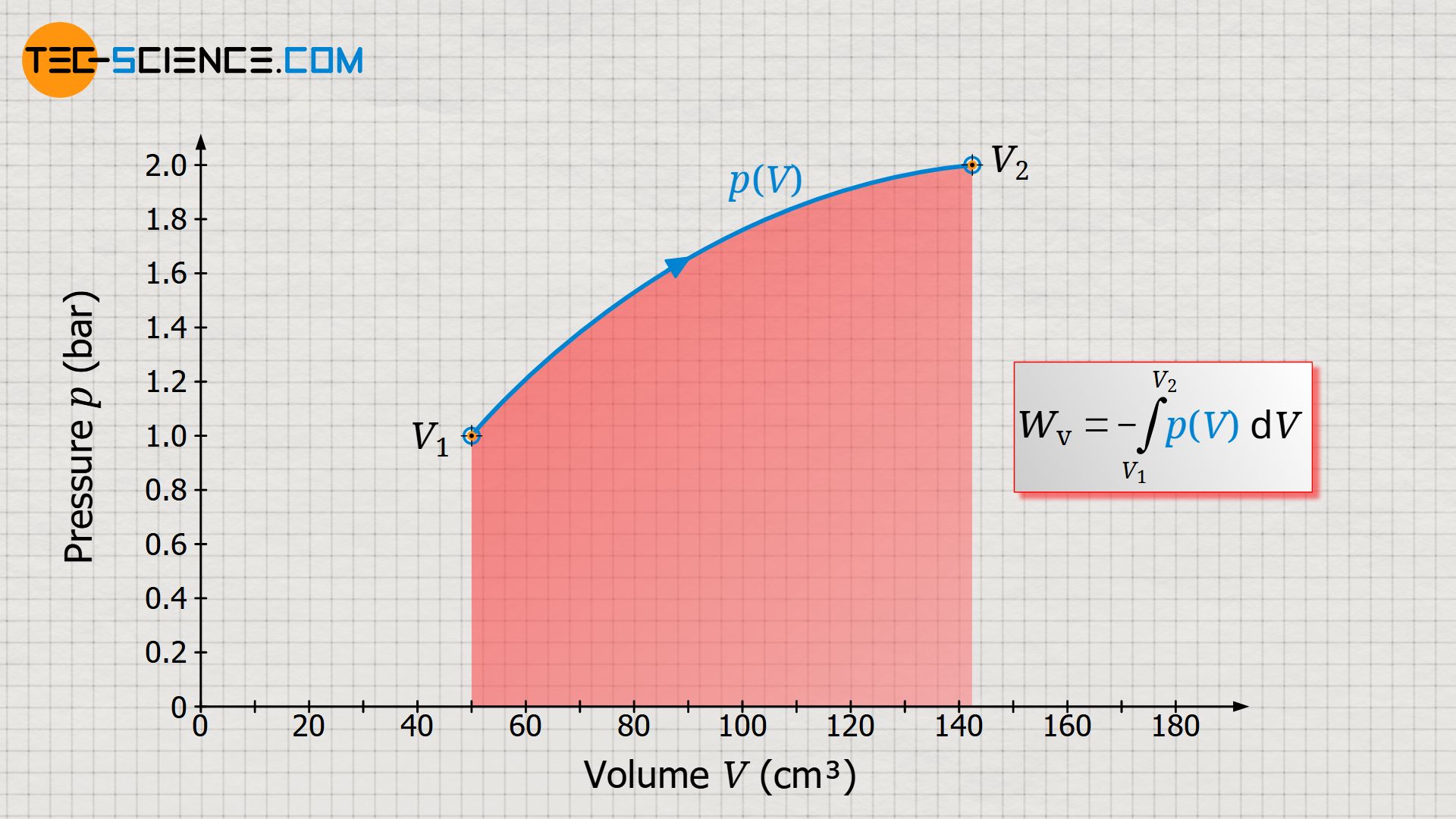
The thermodynamic process is shown in the volume-pressure diagram below. The work done by the gas on the piston corresponds to the pressure-volume work (displacement work) of the gas, which is shown as the area under the curve.

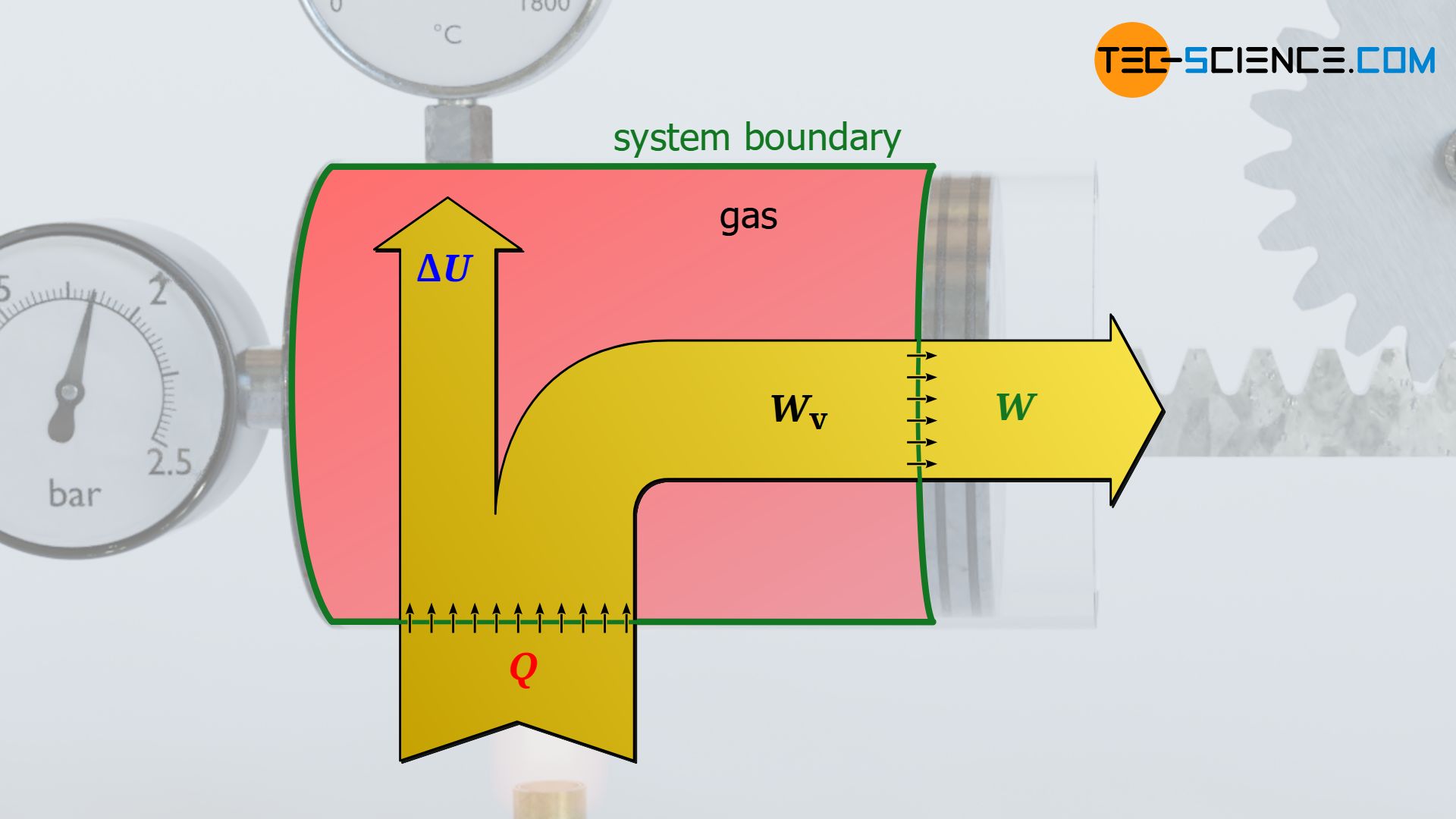
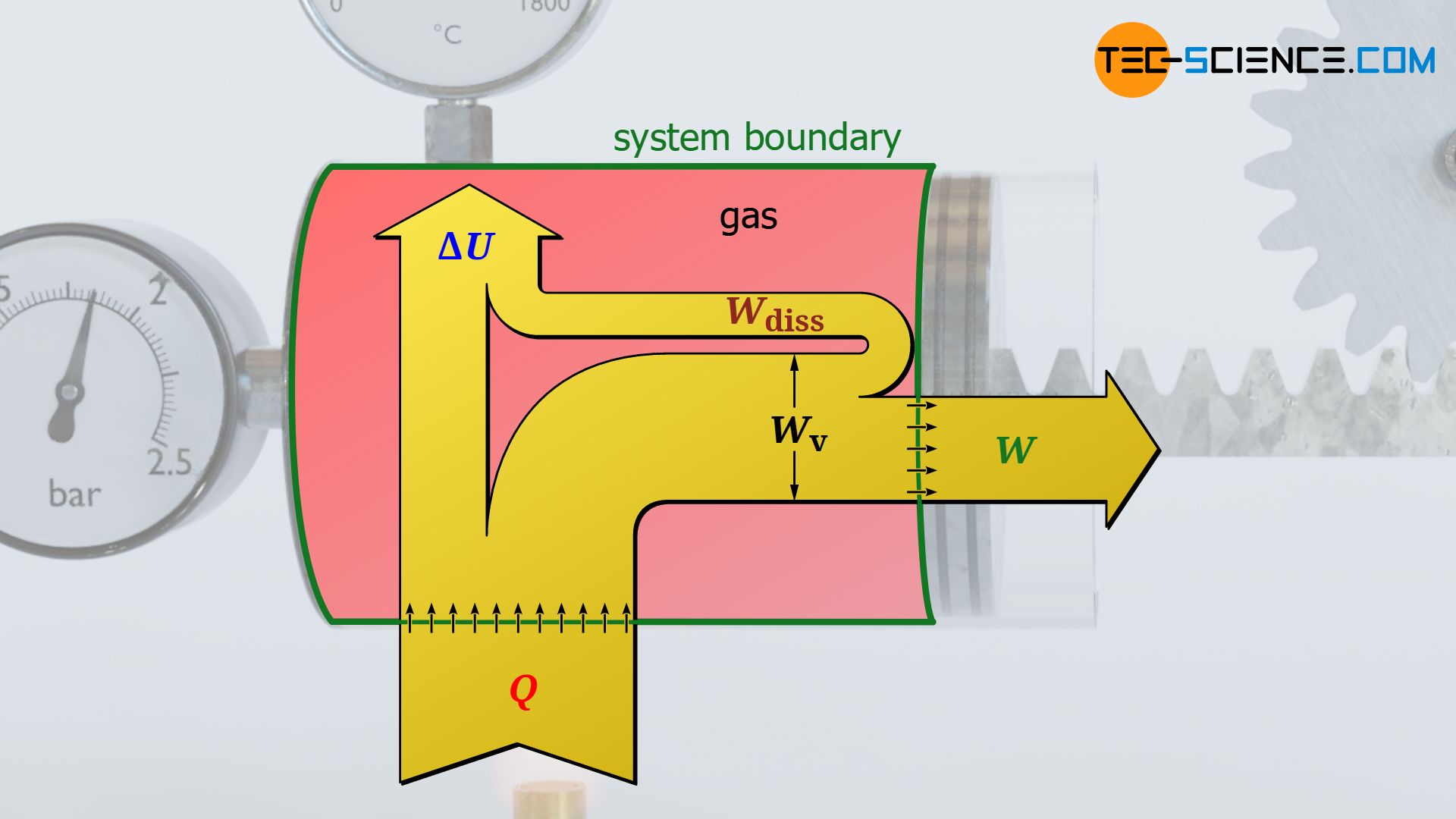
However, the (pressure-volume) work performed by the gas does not necessarily correspond to the work which the system transfers to the surroundings by the piston rod. This will only be the case in a frictionless process. If, on the other hand, friction occurs between the piston and the cylinder wall, not all of the displacement work done by the gas will be transferred to the surroundings. Such a process will be considered in more detail in this article.

Pressure-volume work ≠ work transferred across the boundary

If there is friction between the piston and the cylinder wall, the gas pressure does push the piston while performing pressure-volume work that results as the area under the curve in the volume-pressure diagram. However, this work is not completely transferred to the piston rod across the boundary, since part of the pressure-volume work is dissipated by friction. The system therefore no longer transfers the entire pressure-volume work W_v done by the gas across the system boundary to the surroundings, but only that part minus the frictional work W_diss:

\begin{align}
\label{1}
&\boxed{W = W_\text{v} + W_\text{diss}} ~~~\text{work transferred to the surroundings across the system boundary}
\end{align}

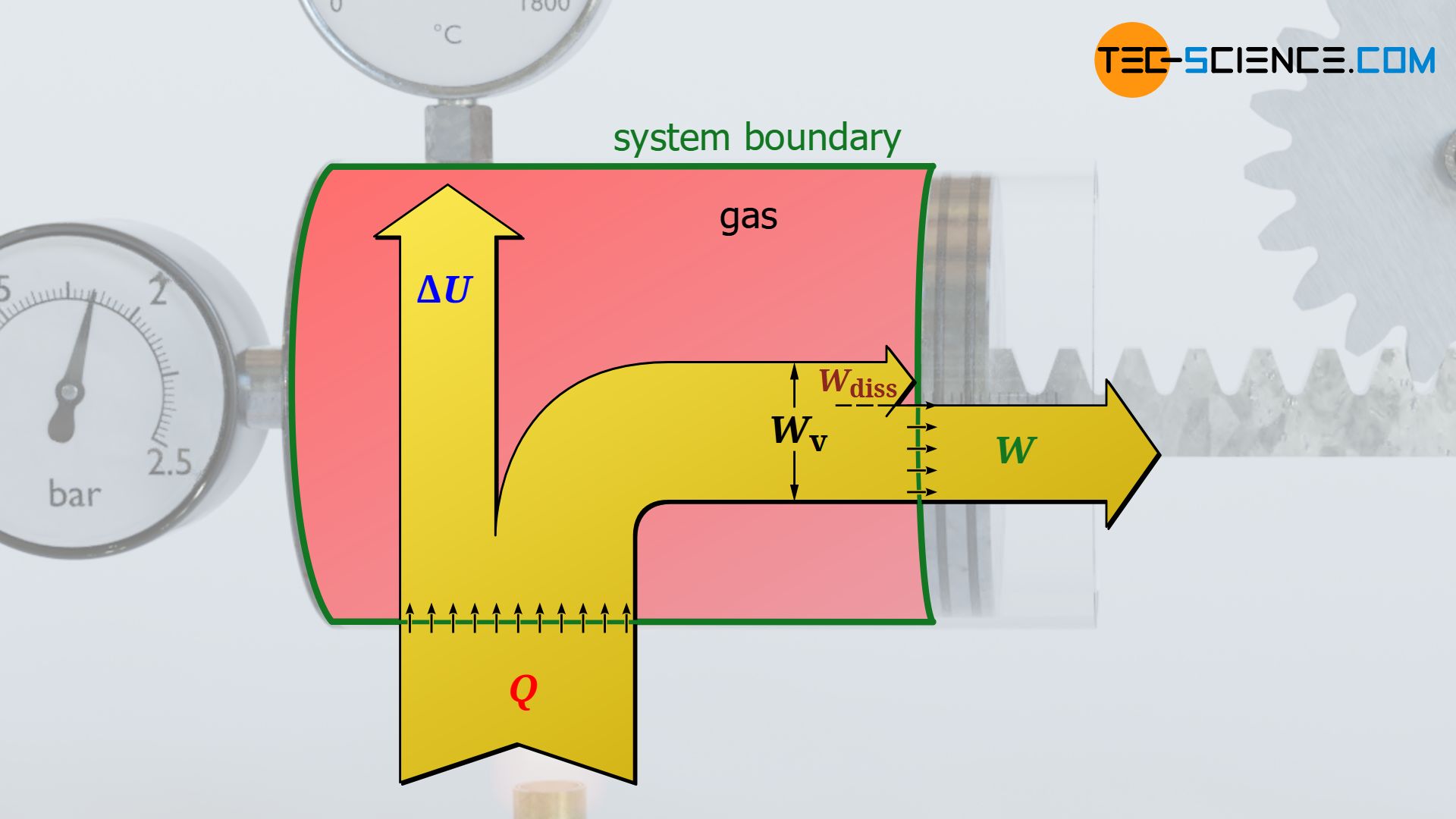
At this point one should not be confused by the plus sign in the equation! This results only due to the sign convention. During an expansion, the negative pressure-volume work W_v is reduced in amount by the positive friction work W_diss. Thus, only a smaller amount of work (W<0) will be transferred across the boundary.

Increase of internal energy by dissipation of energy

So, if the gas in the frictional case yields a greater pressure-volume work than is transferred across the boundary, where does the rest, i.e. the frictional work W_diss go?

The frictional work ultimately leads to an increase in internal energy of the system. In practice, this can be seen from the fact that processes with friction are associated with greater temperatures than frictionless processes. Note that for ideal gases, the internal energy is directly linked to the temperature (see the article Calculating the internal energy for ideal gases). Thus, a higher internal energy always means a higher temperature!

Maybe, one would say at this point:

“Friction is converted to heat, resulting in higher temperatures.”

This statement is not technically correct. Technically correct one would have to say that the friction work benefits the internal energy of the gas and thus causes a temperature increase! The friction work is dissipated into internal energy. Therefore, the friction work is generally denoted by W_diss.

Note that the increased internal energy in processes with friction always results in higher temperatures and thus higher pressures. Accordingly, the pressure level increases during the expansion and thus leads to a larger pressure-volume work compared to the frictionless case (compare the areas under the curves for the two processes, which illustrate the pressure-volume work in each case).

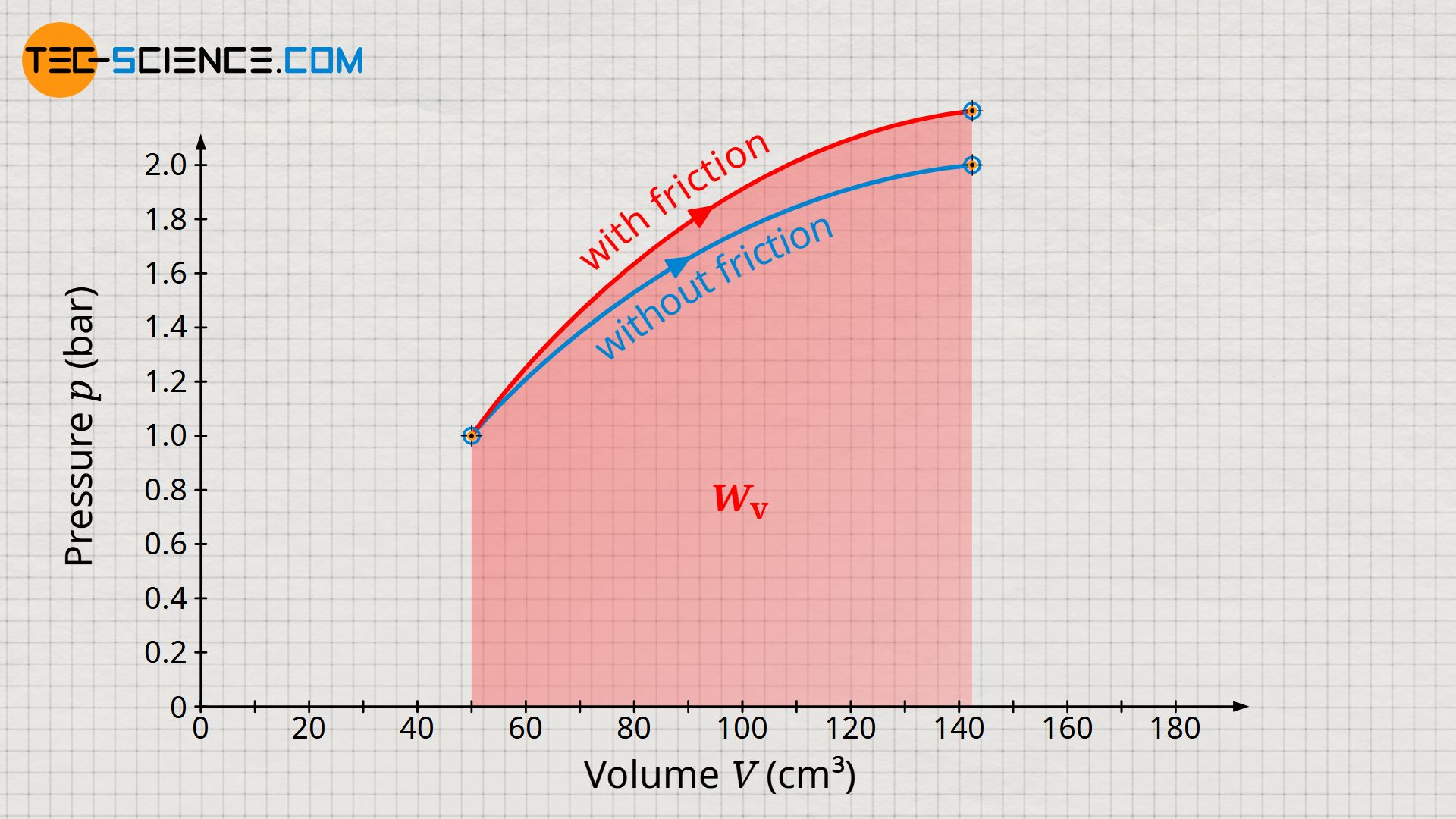
The greater pressure-volume work in the case of an expansion process with friction should not obscure the fact that less work is actually transferred across the system boundary! Only the pressure-volume work minus the dissipated energy is transferred across the system boundary. In spite of the higher pressure-volume work, a smaller amount of work is transferred to the surroundings!

Expansion vs. compression

Equation (\ref{1}), by the way, is valid not only for a thermodynamic process where the gas expands but also for a process where the gas is compressed. This equation expresses that in the case with friction more work W>0 must be applied to the system. Because then not only the gas volume must be compressed against the gas pressure (pressure-volume work W_v>0), but also friction must be compensated (W_diss>0). The positive pressure-volume work and positive friction work thus result in a larger boundary work W>0 that must be done on the system. Here, too, the dissipated energy W_diss benefits the internal energy, resulting in a higher temperature compared to the frictionless case.

These considerations make clear that dissipation of energy always increase the internal energy of a gas; independent of whether the gas expands or the gas is compressed. Dissipation energies therefore always have positive values!

Note that in general, therefore, a distinction must always be made between:

• the pressure-volume work W_v as work done inside the system on the gas or by the gas and
• the work W that actually enters or leaves the system via the system boundary (boundary work).

First law of thermodynamics

The first law of thermodynamics basically only makes a statement about the energy flows across the system boundary, i.e. the heat Q and the work W (not the pressure-volume work inside the system!):

\begin{align}
\label{0}
&\boxed{W + Q = \Delta U} ~~~\text{applies in general} \\[5px]
\end{align}

However, the first law of thermodynamics (\ref{0}) can also be expressed by the pressure-volume work W_v and the dissipated energy W_diss when using equation (\ref{1}):

\begin{align}
\label{2}
&\boxed{W_\text{v} + W_\text{diss} + Q = \Delta U} ~~~\text{applies in general} \\[5px]
\end{align}

Note that only when friction is neglected, i.e. without dissipation of energy (W_diss=0), the pressure-volume work W_v actually corresponds to the boundary work W done by or on the system:

\begin{align}
\label{3}
&\boxed{W_\text{v} + Q = \Delta U} ~~~\text{applies only for processes without friction} \\[5px]
\end{align}